LBM binary mixtures for simulating NMR measurements
- College of Geophysics and Information Engineering, China University of Petroleum, Beijing, China
A lattice Boltzmann binary mixtures method is proposed to simulate the NMR response of fluids in this abstract. Numerical simulation of NMR with classic Monte Carlo random walk method does not consider the interaction between the molecules and the probability distribution field caused by flow. Lattice Boltzmann probability distribution field calculation can be used to acquire the precise NMR simulation results to reproduce the effects of Fick diffusion in the presence of external inhomogeneous background magnetic fields in a Nano pore or throat. CPMG pulse sequence was employed to acquire NMR data. After prepolarization, the liquid in the probe is reversed by the π/2 pulse. Mxy distribution profile at certain time step can be simulated with diffusion and bulk relaxation [1] . The particles whose magnetization vector have been reversed to the xy plane will be miscible with the rest particle, where a Shan-Doolen [2] model was used to describe inter-diffusion factor D:
D=-J1/ (ρtotal ∇ρ1 )
In addition, Shan-Doolen model accurately reproduces spatial distribution of the magnetization vector stemming with Bloch's equation. LBM binary mixtures simulating approach is employed to generate Mxy and Mz imaging to improve the characterization of nano-pore structures and saturated fluids [3] . The intrinsic bulk and surface relaxation times affect the time-space evolution of the macroscopic magnetization of a given volume of fluid. Bloch-Torrey's equation in the rotating frame is:
dM/dt=DB ∇2 M+ γB ×M -(Mx/T2)-(My/T2)-(Mz/T1) +M0/T1
Calculated Mxy image in a Poiseuille flow as shown in Fig.1 and Fig.2.

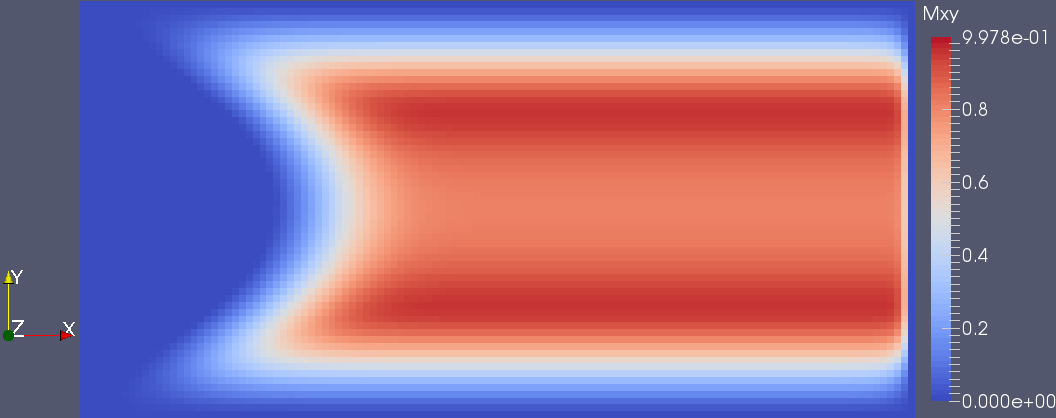
- [1] H. Torrey, (1956), Bloch equations with diffusion terms, APS, Phys. Rev., 563-565, 104
- [2] 2. Xiaowen Shan, Gary Doolen, (1996), Diffusion in a multi-component Lattice Boltzmann Equation model, APS, Phys. Rev., 3614, 54
- [3] K.R. Brownstein, C.E. Tarr,, (1979), Importance of classical diffusion in NMR studies of water in biological cells, APS, Phys. Rev., 19
